Completing the Square: Finding the Vertex
Purplemath
The parabola is the curve that you get when you graph a quadratic equation of the form y = ax2 + bx + c. You've graphed these curves, and you've probably been introduced to the vertex, which is the uppermost (or lowermost) point on the parabola (depending on the direction in which the parabola opens).
But now you're being asked to find the vertex specifically, and — of course! — they're making the vertex hard to find from the graph. To find the coordinates of the vertex, you're going to have to "complete the square".
Content Continues Below
Why do I have to complete the square?
Completing the square, when dealing with a parabola's quadratic equation, reformats that quadratic equation into the vertex form; from this vertex form, you can read off the coordinates of the vertex of the parabola. When those coordinates are messy — say, (−¾, 3.7) — completing the square is the only way to be sure of the exact x- and y-values for the vertex.
Can't I use my calculator to find the vertex?
Advertisement
Yes, you can probably use your graphing calculator to get at least close to the values, but simple fractional values like will often be stated as non-terminating decimals; in this case, the screen would show "0.1428571", which isn't very helpful, especially when your answers are required to be "exact".
Also, your instructor may decide to have at least a portion of the test be no-calculators [and no phones], so you probably do need to know how to do this by hand.
What is the vertex form of a parabola's quadratic?
The vertex form of a parabola's quadratic equation looks like this:
y = a(x − h)2 + k
When the equation is reformatted as above, the point (h, k) is the vertex.
Affiliate
The a in the vertex form is the same a as in y = ax2 + bx + c; that is, both of the a's have exactly the same value.
The sign on a (plus or minus) tells you whether the quadratic's parabola opens up or opens down. Think of it this way: A positive a draws a smiley face, and a negative a draws a frowny face. (Yes, it's a silly picture to have in your head, but it makes is very easy to remember how the leading coefficient works.)
Content Continues Below
When they're wanting you to find the exact coordinates of the vertex of a parabola, they don't usually give you the quadratic in vertex form; instead, they usually give you the quadratic in the regular y = ax2 + bx + c format. How do you convert from the regular format to the vertex format? By using the technique of completing the square. Here's an example:
- Find the vertex of y = 3x2 + 2x − 1
This is my original equation:
y = 3x2 + 2x− 1
First, I'll move the loose number over to the other side of the equation, with the y:
y + 1 = 3x2 + 2x
Now I'll factor out whatever is multiplied on the squared term, keeping in mind that:
"Factor" does not mean to "make disappear" or "divide off onto the other side"; "factor" means "divide out front".
So I'll just pull that 3 out front, giving me:
For the purposes of completing the square, I'm going to create space on the left-hand side with the y, and, if a is anything other than simply 1, I'll also put a copy of a in front of this space. I'll need this space and the copy of a to keep my equation "balanced".
Now I'll take half of the coefficient of the linear x-term from inside the right-hand side parentheses (that is, I'll divide that coefficient by two), not forgetting its sign, square the result, and add it to both sides inside the parentheses. (This is why the parentheticals were created in the first place.)
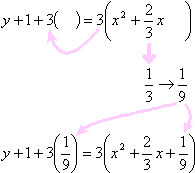
On the left-hand side of the equation, I'll multiply out the "a times the squared coefficient" part on the left-hand side, and convert the right-hand side to squared form. (This is where I use that sign that I kept track of earlier, putting that sign in the middle of the squared expression.)
I'll simplify some more, as necessary:
Now I'll move the loose number from the left-hand side back over to the right-hand side:
To be thorough, I'll reformat into vertex form (which is a matter of making sure the signs match the vertex form's formula), and read off the vaues of h and k.
In this case, since a = 3 (which is a positive number), then this is a right-side-up parabola (that is, it's an upward-opening curve), and the vertex, , is the lowest point on the graph.
You may be wondering why I went to the trouble of reformatting the equation to "proper" vertex form:
I did this because the formula for the vertex form is:
y = a(x − h)2 + k
I wanted to make very clear to myself that the value that was subtracted from x to result in the binomial was a negative value. Because, to get a "plus" in the simplified form, I had to have subtracted a "minus":
The two minuses cancelled to give me the plus inside the parenthetical expression.
Turning to the loose number tacked on after the squared parenthetical, I see that it is subtracted from the parenthetical. To make this fit the vertex form of the quadratic, I converted the subtraction to the addition of a negative, because:
Affiliate
Warning: It's easy to confuse yourself at this final stage of the process, by trying to read off the vertex as "(h, k) = (whatever number is inside the squared part, whatever the other number is) ", without noticing the fact that the h-part is subtracted and the k-part is added.
(This is part of why I wandered through that long "aside" above, about why the vertex being (h, k) made sense, if you thought about it. Because if you are aware of the logic behind (h, k) being the vertex, then it's generally easier for you to keep the formula straight.)
If you take care to ensure that you have your quadratic completely converted to vertex form by being careful of the signs, then you'll be able to avoid one of the most commonly-made mistakes for these problems (namely, having the wrong signs on the coordinates of the vertex). Make sure you practice this until you can consistently interpret your results correctly.
By the way, did you notice that the vertex coordinates weren't whole numbers? Instructors are starting to figure out that students are guessing the vertex from the pretty pictures in their graphing calculators, and they know that students often have the idea that all answers are always either whole numbers or "neat" fractions like one-half. For instance, if the calculator screen estimates a vertex as being at (0.48, 0.98), many students will assume that the answer must "really" be (0.5, 1), instead of, say, .
So, in order to check that students really do know how to find the vertex (and not just guess a decimal approximation from a picture), teachers are giving more complicated exercises. If you have been told that you should know this technique for finding the vertex, rest assured that your teacher has ways of checking whether you have really learned this. Don't plan on using calculator cheats.
What are the steps for completing the square?
To take a general quadratic equation and reformat it so you can find the vertex of the associated parabola, follow these steps:
- Collect the x-containing terms on the right-hand side of the "equals" sign, with the y and any loose numbers on the left-hand side of the "equals".
- If any number a ≠ 1 is multiplied onto the x2 term, factor this out and move it in front of the parenthetical containing the x-terms.
- Rewrite each side of the equation to add room for completing the square.
- On the left-hand side with the y, add an empty parenthetical, multiplied by the factored-out value a, if applicable.
- On the right-hand side, add extra space inside the parenthetical. (This parenthetical already has the a multiplied onto it, if applicable.)
- Take the coefficient of the linear x-term — along with its sign! — and multiply by one-half.
- Square this new value, and add it inside the parentheticals on either side of the equation.
- Convert the right-hand side to a squared binomial (multiplied by a, if applicable).
- Move any loose numbers from the left-hand side over to the right-hand side.
- Make sure that your signs inside the vertex form are correct, and then read off the value of the vertex.
URL: https://www.purplemath.com/modules/sqrvertx.htm
You can use the Mathway widget below to practice converting to vertex form. (Or skip the widget, and continue with the next page.) Try the entered exercise, or type in your own exercise. Then click the button and select "Find the Vertex Form" to compare your answer to Mathway's.
Please accept "preferences" cookies in order to enable this widget.
(Click "Tap to view steps" to be taken directly to the Mathway site for a paid upgrade.)
Select a Course Below
Standardized Test Prep
Homeschool Math
© 2024 Purplemath, Inc. All right reserved. Web Design by ![]()