Special Angle Values: Worked Examples
Purplemath
-
Find the values of sin(45°), cos(45°), and tan(45°).
Using the basic reference triangle:
![45° triangle, with sides having lengths sqrt[2] and hypotenuse having length 2; the right angle is at the right of the base; the base angle is at the left of the base; the opposing angle is to the upper right](trig/trig05.gif)
...I can read off the values, and they're already in "rationalized denominator" form:
-
Evaluate csc(225°)
From what I've learned about trig ratios, I know that the cosecant is the reciprocal of the sine. I can see that the angle value they've given me can be expressed as:
225° = 180° + 45°
So I know that I'm in the third quadrant, where sine is negative. In particular, I'm forty-five degrees in, so I'll be using the sine of forty-five degrees, from the first quadrant, and then applying the cosecant and quadrant information:
-
Find the lengths of the legs of the 45-45-90 triangle having as its hypotenuse a line segment of length 4 cm.
First, I'll quickly draw the triangle they've given me, labelling the legs with "L":
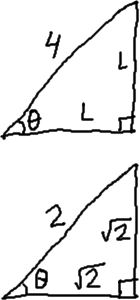
Comparing the triangle they've given me (the first triangle above) to the similar reference triangle (the second triangle above), I can set up a proportion in order to figure out the length of each leg of the new triangle. My ratios will have the new triangle's info on top in the fractions, and the reference triangle's info on the bottom. The length of the other leg, L, is found by:
Because a 45-45-90 triangle is isosceles, this gives me the lengths of both of the legs. This value is the length that they're seeking, so my answer, including the units, is:
legs' length: cm
You'll note that my triangles, in my working above, aren't very pretty. In your scratch-work, you don't have to be particularly neat. I was using the triangles mostly as placeholders, so I could keep track of how this particular triangle's info related to that of the reference triangle. It's perfectly okay to be messy like this. Don't stress about making your placeholder triangle be perfect.
By the way, when you reach calculus, they won't usually care so much about whether or not there are radicals in the denominator. At that stage, it may be simpler to use the following as your reference triangle:
![45° triangle, with sides having lengths 1 and hypotenuse having length sqrt[2]; the right angle is at the right of the base; the base angle is at the left of the base; the opposing angle is to the upper right](trig/trig09.gif)
Either reference triangle will give you the same results.
-
Evaluate sin(30°), cos(150°), and tan(330°).
The first value is easy. I'll do a quick-n-dirty sketch of a 30-60-90 triangle, with the 30° angle at the left:
![30-60-90 triangle, with angle at left labelled 30, the right angle at the right, the base (the adjacent side) labelled sqrt[3], the height (the opposite side, on the right) labelled 1, and the hypotenuse labelled 2](trig/trig10.gif)
Now I can read the value from the picture:
The second angle can be stated as:
150 = 180 − 30
So this angle is thirty degrees into the second quadrant, if I'm backing up from the negative x-axis. The cosine is negative in the second quadrant. So I'll use the first-quadrant value of cos(30°), being , but with the opposite sign:
The last angle can be stated as:
330 = 360 − 30
So this angle is thirty degrees into the fourth quadrant, if I'm moving backwards from one full rotation. The tangent is negative in the fourth quadrant, so I'll use the first-quandrant value, but with the opposite sign:
Then my complete answer is:
-
Evaluate sin(60°), csc(240°), and cot(120°)
First, I'll do a quick-n-dirty sketch of my reference triangle:
![30-60-90 triangle, with the 60-degree triangle at bottom left and labelled 60, the right angle at bottom right; the base (the adjacent side) is labelled 1, the altitude (the opposite side, on the right) is labelled sqrt[3], and the hypotenuse is labelled 2](trig/trig11.gif)
The first angle is easy; I'll just read the value off my triangle:
The second angle can be stated as:
240 = 180 + 60
So this angle is sixty degrees into the third quadrant. The cisecant is the reciprocal of the sine, and the sine is negative in the third quadrant. So I'll use the first-quadrant value of sine, flipped upside down, and with the opposite sign:
The third angle can be stated as:
120 = 180 − 60
So this angle is sixty degrees into the second quadrant, if I'm backing up from the negative x-axis. The cotangent is the reciprocal of the tangent, and the tangent is negative in the second quadrant. So I'll use the first-quadrant value of tangent, flipped upside-down, and with the opposite sign:
Then my complete answer is:
-
The side opposite the 30° angle in a right triangle has length 2 sqrt[3] ft. Find the lengths of the other two sides of the triangle.
It might seem like I don't have enough information, but I do, because all 30-60-90 triangles are similar. So I can start with sketches of my reference triangle, and the triangle they've given me here:
![the 30-degree reference triangle sketched first; the exercise's triangle follows below the other triangle, with the altitude (the opposite side, on the right) labelled 2×sqrt[3], the base (the adjacent side) labelled x, and the hypotenuse labelled h](trig/trig12.gif)
I can find the lengths of the other sides by setting up and solving proportions. First, I'll find the length of the base, which I've labelled "x in my picture:
I can find the length of the hypotenuse in the same way:
Then my answer, together with the units, is:
leg: 6 ft
hyp.: ft
Note: If the above answers were meant to be used in a word problem, or in "real life", we'd probably want to plug them into a calculator in order to get more-helpful decimal approximations. However, in your math classes, unless you're told to approximate, you should assume that they're wanting the "exact" forms shown above.
© 2024 Purplemath, Inc. All right reserved. Web Design by 