Solving Proportions: Word Problems
Purplemath
Many "proportion" word problems can be solved using other methods, so they may be familiar to you. For instance, if you've learned about straight-line equations, then you've learned about the slope of a straight line, and how this slope is sometimes referred to as being "rise over run".
But that word "over" gives a hint that, yes, we're talking about a fraction. And this means that "rise over run" can be discussed within the context of proportions.
-
You are installing rain gutters across the back of your house. The directions say that the gutters should decline inch for every four feet of lateral run. The gutters will be spanning thirty-seven feet. How much lower than the starting point (that is, how much lower than the high end) should the low end of the gutters be?
Rain gutters have to be slightly sloped so the rainwater will drain toward and then down the downspout. As I go from the high end of the guttering to the low end, for every four-foot length that I go sideways, the gutters should decline [be lower by] one-quarter inch. So how much must the guttering decline over the thirty-seven foot span? I'll set up the proportion, using "d" to stand for the distance I'm needing to find.
There is a variable in only one part of my proportion, so I can use the shortcut method to solve.
![multiplying 37 and 1/4 (diagonally on the green arrow), and then dividing by 4 (hooking back on the purple arrow), to get d = [(37)(1/4)]/4](ratio/ratio35.gif){kind=small}
For convenience sake (because my tape measure isn't marked in decimals), I'll convert this answer to mixed-number form:
The lower end should be inches lower than the high end.
As is always the case with "solving" exercises, we can check our answers by plugging them back into the original problem. In this case, we can verify the size of the "drop" from one end of the house to the other by checking the products of the means and the extremes (that is, by confirming that the cross-multiplications match) of the completed proportion:
Converting the "one-fourth" to "0.25", we get:
(0.25)(37) = 9.25
(4)(2.3125) = 9.25
Since the values match, then the proportionality must have been solved correctly, and the solution must be right.
-
Biologists need to know roughly how many fish live in a certain lake, but they don't want to stress or otherwise harm the fish by draining or dragnetting the lake. Instead, they let down small nets in a few different spots around the lake, catching, tagging, and releasing 96 fish. A week later, after the tagged fish have had a chance to mix thoroughly with the general population, the biologists come back and let down their nets again. They catch 72 fish, of which 4 are tagged. Assuming that the catch is representative, how many fish live in the lake?
As far as I know, biologists and park managers actually use this technique for estimating populations. The idea is that, after allowing enough time (it is hoped) for the tagged fish to circulate throughout the lake, these fish will then be evenly mixed in with the total population. When the researchers catch some fish later, the ratio of tagged fish in the sample to untagged is representative of the ratio of the 96 fish that they tagged with the total population.
I'll use " f " to stand for the total number of fish in the lake, and set up my ratios with the numbers of "tagged" fish on top. Then I'll set up and solve the proportion:
Because the variable is in only one part of the proportion, I can use the shortcut method to solve.
![multiply 96 and 72 (along the green arrow), then divide by 4 (hooking back on the purple arrow), to get d = [(96)(72)]/4](ratio/ratio37.gif){kind=small}
This tells me that the estimated population is:
about 1,728 fish
Another type of "proportion" word problem is unit conversion, which looks like this:
-
How many feet per second are equivalent to 60 mph?
To complete this exercise, I will need conversion factors, which are just ratios. (If you're doing this kind of problem, then you should have access — in your textbook or in a handout, for instance — to basic conversion factors. If not, then your instructor is probably expecting that you have these factors memorized.)
I'll set everything up in a long multiplication so that the units cancel:
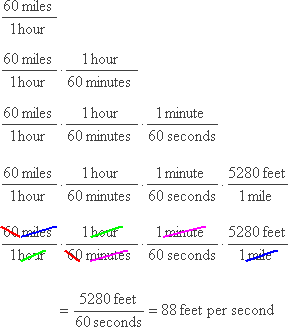
Then my answer is:
88 feet per second
Take note of how I set up the conversion factors for my multiplicate (above) in not-necessarily-standard ways. For instance, one usually says "sixty minutes in an hour", not "one hour in sixty minutes". So why did I enter the hour-minute conversion factor (in the second line of my computations above) as "one hour per sixty minutes"?
Because doing so lined up the fractions so that the unit of "hours" in my conversion factor would cancel off with the "hours" in the original "60 miles per hour". This cancelling-units thing is an important technique, and you should review it further if you are not comfortable with it.
-
A particular cookie recipe calls for 225 grams of flour for one batch of thirty cookies. Jade would like to make as many cookies as possible for the upcoming block party, and flour is his only constraint (he's got loads of sugar, eggs, etc). If he has 1.206 kilograms of flour, and assuming that all cookies are the same size, approximately how many cookies can he make? (Round to an appropriate whole number.)
I've got two elements here for my proportion: grams of flour and number of cookies. I got to "grams" first when reading the exercise, so I'll put "grams" on top in my proportion.
Since the relationship is given to me in terms of grams, not kilograms, I'll need to convert Jade's on-hand measure to "1,206 grams, also. I'll use "c" to stand for the number that I'm trying to figure out for "cookies".
Since I have an unknown in only one spot in this proportion, I can use the shortcut method to solve.
![multiply 1206 and 30 (along the diagonal green arrow), divide by 225 (hooking back along the purple arrow), to get d = [(1206)(30)]/225](ratio/ratio54.png){kind=small}
Ohhh! Now I see why the instructions said to round to an "appropriate" whole number: Jade can only make whole cookies; the "point-eight" of a cookie will be an undersized niblet that he'll eat before heading to the party.
While normally I'd round this number up to get my whole-number answer, in this case I need to round down; in other words, in this context (namely, of all the cookies being the same size), I have to ignore the fractional portion (that is, the point-eight decimal part) to get the desired answer.
160 cookies
-
Kumar lives in Croatia, and is visiting relatives in India. The current exchange rate is one Euro (€1) to 80.45 Indian rupees (₹80.45). He wants to buy a gift for them, which costs ₹3,759. How many Euros will this gift cost him? (State your answer accurate to two decimal places.)
They've given me an exchange rate, which is, effectively, just another conversion factor, like the "miles per hour" exercise above. So I'll set up my proportion, with Euros on top, and will use e to stand for the number of Euros he'll need.
I'll use the shortcut method to solve:
Rounding to two decimal places, Kumar will be spending:
€46.72
Other than for the rate-conversion exercise above, we've been able to solve all of the proportions by the shortcut method. You will likely find this to be the case in your homework, also. But it is always possible that you'll get a question where you'll be better off using cross-multiplication instead.
-
George has heard from two different sources about the pay range at a particular company. One source says that the ratio of lowest pay to highest pay is 3 : 7. The other source says that the top earner annually makes about $57,000 more than the lowest earner. What are the approximate salaries for the highest and lowest earners? (Round to the nearest thousand.)
I know that the ratio is 3 : 7, so I'll be using the fraction 3/7 for one side of my proportion. If the lowest pay rate, in thousands of dollars, is L, then the highest is L + 57. My proportion is:
Because there are variables in two of the parts of this proportion, the shortcut method won't be as useful as cross-multiplication to clear all the fractions. So I'll cross-multiply:
Remembering that I dropped the trailing zeroes and am counting by thousands, the above number means that the lowest salary is (rounded to the nearest thousand) approximately $43,000. Then the highest salary, being around $57K more, is approximately $100,000.
lowest: $43,000
highest: $100,000
© 2024 Purplemath, Inc. All right reserved. Web Design by 