Symmetry About a Point
Purplemath
In addition to finding lines (axes) of symmetry, you can also look for points of symmetry.
A point of symmetry is a point that represents a "center" of sorts for the figure. For any line that you draw through the point of symmetry, if this line crosses the figure on one side of the point, the line will also cross the figure on the other side of the point, and at exactly the same distance from the point
For instance, a figure-eight has a point of symmetry in the middle, where the lines cross (shown below by the blue dot):
Content Continues Below
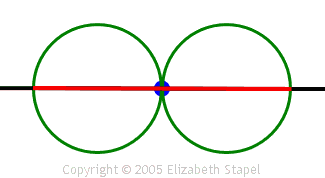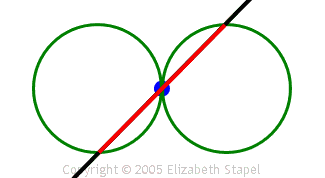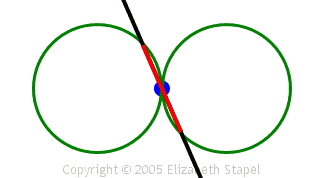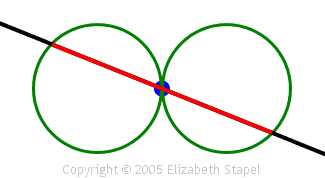
(The above graphic is animated on the "live" site.)
At each stage of the rotation, either the line through the point doesn't intersect the figure at all (so the line is all black), or else the distance along the line to the figure in one direction is the same as the distance along the line to the figure in the other direction (shown by the red portion of the black line).
For an hyperbola, the center is the point of symmetry:
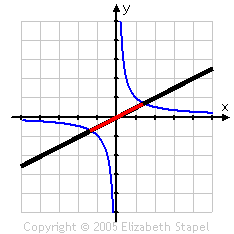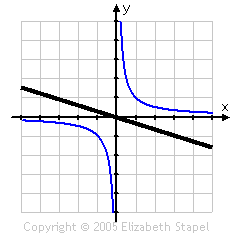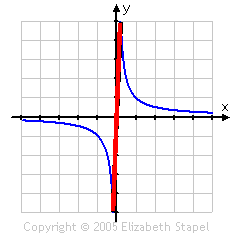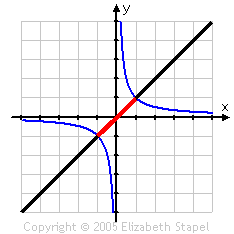
(The above graphic is animated on the "live" site.)
As you can see from the hyperbola, a point of symmetry doesn't have to be a point on the figure; it can, as it does here, lie outside the figure or graph. Also, in this case, the point of symmetry happens to be the origin. That will not always be true. For instance, if the hyperbola were shifted at all, then its point of symmetry would be moved off the origin.
Content Continues Below
You can also view points of symmetry as being points about which you can rotate the shape 180° (with the resulting, rotated graph looking identical to the original graph), as shown below with the hyperbola:
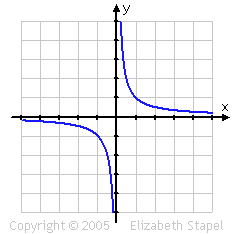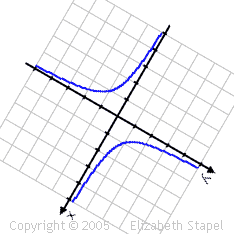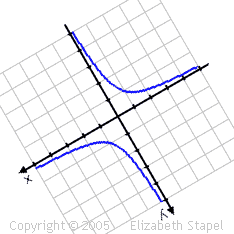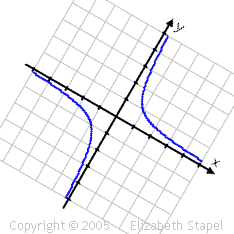
(The above graphic is animated on the "live" site.)
...and the figure-eight:
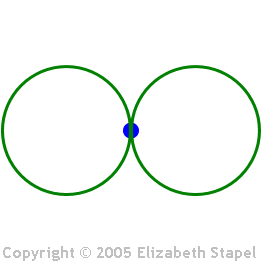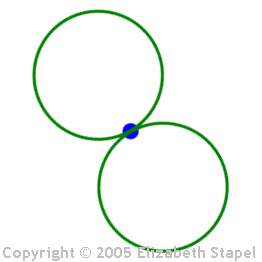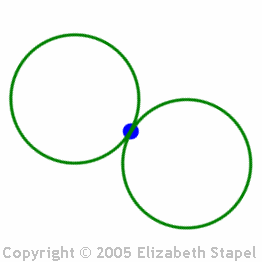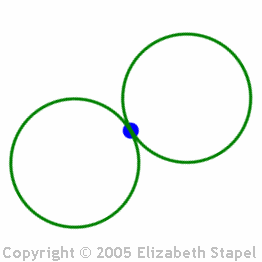
(The above graphic is animated on the "live" site.)
Affiliate
On the previous page, we saw that lines of symmetry of a graph need not be either of the x- or y-axis. In the same way, points of symmetry need not be at the origin. For instance:
point of symmetry:
not at the origin

We also saw, on the previous page, that the axis of symmetry might not cross or touch the graph. In the same way, points of symmetry need not lie on the graph. For instance:
point of symmetry:
not on the graph
When working with functions, you'll be asked to check if the function is "odd" which means you'll be checking if the graph is symmetric about the origin. The only other point-symmetry you'll likely be asked about will be the centers of conics.
The graph of y = x3 is shown below:
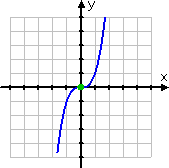
The point of symmetry is the green point, which is at the origin. In this context, you'd likely be asked something like, ""Is the function odd?", and you'd answer "Yes."
On the other hand, the graph of y = (x − 1)3 − 2 is shown below:
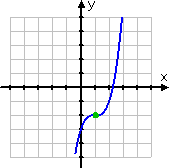
The point of symmetry in the above graph is at the same place on the curve but, because the curve was shifted, that point of symmetry is no longer at the origin; instead, it is now at (1, −2). If you were asked, "Is this function odd?", you would answer, "No", because the point of symmetry is not at the origin. However, if you were just asked for the point of symmetry, you would answer, "(1, −2)".
Affiliate
Affiliate
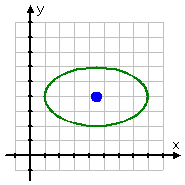
The centers of certain conics (namely, circles, ellipses, and hyperbolas) are points of symmetry. The graph of the ellipse is shown below:
The center of the ellipse is its point of symmetry and, in this case, the point of symmetry is the origin.
On the other hand, the graph of is shown below:
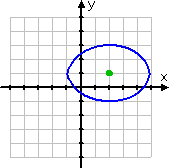
In this case, the point of symmetry is not at the origin, but is instead located at (2, 1).
If you are looking at point-symmetry in the context of "odd" functions, then you'll be asked questions such as, "Is the graph of y = x5 symmetric about the origin?" You would answer, "Yes." But if you were asked, just generally, if a given function or relation has a point of symmetry, you'd be asked something like, "Find the point of symmetry for (x − 3)2 + (y + 2)2 = 1." You'd note, from the equation, that this is a circle, and you'd answer with the coordinates of the center: "point of symmetry: (3, −2)"
URL: https://www.purplemath.com/modules/symmetry2.htm
Select a Course Below
Standardized Test Prep
Homeschool Math
© 2024 Purplemath, Inc. All right reserved. Web Design by ![]()



