Solving Higher-Index Radical Equations
Purplemath
While most ("nearly all"?) of the radical equations you'll be given to solve will involve square roots, you may also see some higher-index equations, as well. They work in pretty much the same way. For instance, if you're given an equation where the radical is a cube root, you'll cube both sides (after isolating the radical) to convert the equation to a polynomial that you can solve.
-
Solve the equation:
Since this is a CUBE root, rather than a square root, I will undo the radical by cubing both sides of the equation, rather than by squaring:
My answer is:
x = 16
You may be wondering why I didn't check my solution. I have to check my solutions for equations where I've squared, or where I've raised both sides of the equation to some other even degree. Why?
Because squaring and the like get rid of minus signs, which can create solutions that don't actually exist. But the solution process in the exercise above involved cubing, which preserves minus signs. This is why I didn't need to check.
(Note: If your instructor wants you to check, and show your check, for every exercise, regardless of the index of the radical(s) involved, then checking every time is "the right way" for that class. If you're not sure what you're supposed to do, ask now, before the next test.)
-
Solve the equation:
I note that the "plus one" on the left-hand side of the equation is outside of the cube root. I'll need to move it over to the right-hand side of the equation before I cube both sides.
This solution involved cubing, not squaring, so I don't (technically) need to check my solution. My answer is:
-
Solve the equation:
Since this is a fourth root, I'll raise both sides to the fourth power. (Also, since this is an even-index root, I'll definitely need to check my answer.)
The original equation was a fourth root, and the solving process involved my raising both sides of the equation to the fourth power (in particular, to an even power). So I'll have to check my answers. Here's one of the checks:
The left-hand side (LHS) ended up equalling the right-hand side (RHS), so this solution checks. (I'll leave the check of the other solution for you.) My answer is:
By the way, graphing does indicate that both solutions are valid. If we regard the left- and right-hand sides of the original equation as being their own functions, we get:
Graphing, we get this:
It's kinda hard to see, so we zoom in on that middle section until we're sure that we can see that there are two intersection points (and thus that there are two solutions to the original equation):
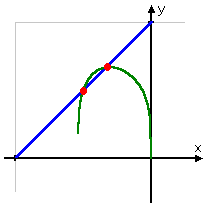
If you're wondering about why the graph of the radical side is split into three pieces, it's because we can't have negatives inside a fourth root. The graph only exists where the argument of the radical, being the polynomial x4 + 4x3 − x, is non-negative. This occurs in three pieces, being where the graph of the argument is at or above the x-axis:
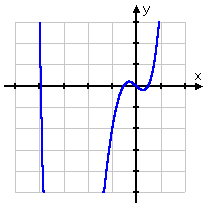
Don't be shy about using your graphing calculator to confirm (or correct) your solutions.
You may or may not be required to show solutions graphically, but if you have a graphing calculator (so drawing the graphs is just a matter of quickly punching a few buttons), you can use the graphs to check your work on tests.
Since cube roots can have negative numbers inside them, you don't tend to have the difficulty with them regarding checking the answers that you did with square roots. However, you will have those difficulties with fourth roots, sixth roots, eighth roots, etc; namely, any even-index root. Be careful, and remember to check your solutions when the index requires it. And remember that you square (or cube, or whatever) sides of an equation, not the individual terms.
© 2024 Purplemath, Inc. All right reserved. Web Design by 