Horizontal and Vertical Lines

This relationship will become very important when you start working with straight-line equations.

---

Now let's consider those two equations and their graphs.

For the first equation, , the slope was , a positive number. The graph looked like this:

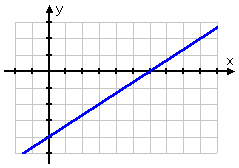
Notice how the line, as we move from left to right along the x-axis, is edging upward toward the top of the drawing; technically, the line is an "increasing" line. And... the slope was positive.

This relationship always holds true: If a line is increasing, then its slope will be positive; and if a line's slope is positive, then its graph will be increasing.

For the second line, y = −2x + 3, the slope was m = −2, a negative number. The graph looked like this:

Notice how the line, as we move from left to right along the x-axis, is edging downward toward the bottom of the drawing; technically, the line is a "decreasing" line. And... the slope was negative.

This relationship is always true: If a line is decreasing, then its slope will be negative; and if a line's slope is negative, then its graph will be decreasing.

---

This relationship between the sign on the slope and the direction of the line's graph can help you check your calculations: if you calculate a slope as being negative, but you can see from the graph of the equation that the line is actually increasing (so the slope must be positive), then you know you need to re-do your calculations. Being aware of this connection can save you points on a test because it will enable you to check your work before you hand it in.

---

So now we know: Increasing lines have positive slopes, and decreasing lines have negative slopes. With this in mind, let's consider the following horizontal line:

Its graph is shown below:

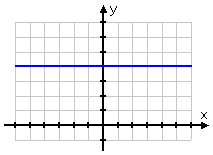
Is the horizontal line edging upward; that is, is it an increasing line? No, so its slope can't be positive. Is the horizontal line edging downward; that is, is it a decreasing line? No, so its slope can't be negative. What number is neither positive nor negative?

Zero!

So the slope of this (and any other) horizontal line should, logically, be zero. Let's do the calculations to confirm this. Using the (arbitrary) points from the line, (−3, 4) and (5, 4), the slope computes as:

This relationship always holds: a slope of zero means that the line is horizontal, and a horizontal line means you'll get a slope of zero.

(By the way, all horizontal lines are of the form "y = some number", and the equation "y = some number" always graphs as a horizontal line.)

---

Now consider the following vertical line:

Its graph is below.

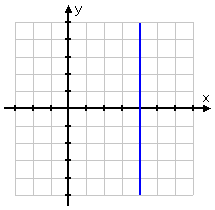
Is the vertical line going up on one end? Well, yes, kind of. So maybe the slope will be positive...? Is the vertical line going down on the other end? Well, again, kind of. So maybe the slope will be negative...?

But is there any number that is both positive and negative? Nope.

Verdict: vertical lines have NO SLOPE. The concept of slope simply does not work for vertical lines. The slope of a vertical line does not exist!

Let's do the calculations to confirm the logic. From the line's graph, I'll use the (arbitrary) points (4, 5) and (4, −3). Then the slope is:

We can't divide by zero, which is of course why this slope value is "undefined".

This relationship is always true: a vertical line will have no slope, and "the slope is undefined" or "the line has no slope" means that the line is vertical.

(By the way, all vertical lines are of the form "x = some number", and "x = some number" means the line is vertical. Any time your line involves an undefined slope, the line is vertical; and any time the line is vertical, you'll end up dividing by zero if you try to compute the slope.)

It is very common for tests to contain questions regarding horizontals and verticals. Don't mix them up!