Conjugates & Dividing by Radicals
Purplemath
Sometimes you will need to multiply multi-term expressions which contain only radicals. This is a situation for which vertical multiplication is a wonderful help.
-
Simplify
This exercise looks ugly, but it's perfectly do-able, as long as I'm neat and precise in my work.
First, I do the multiplication, using the vertical method to keep things straight:
Then I set the original expression equal to the last line from the multiplication above, and complete the calculations by simplifying each term:
-
Simplify:
First I do the multiplication:
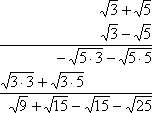
And then I simplify:
Note in the last example above how I ended up with all whole numbers. (Okay, technically they're integers, but the point is that the terms do not include any radicals.) I multiplied two radical binomials together and got an answer that contained no radicals. You may also have noticed that the two "binomials" were the same except for the sign in the middle: one had a "plus" and the other had a "minus".
This pair of factors, with the second factor differing only in the one sign in the middle, is very important; in fact, this "same except for the sign in the middle" second factor has its own name:
Given the radical expression 
, the "conjugate" is the expression 
.
The conjugate (KAHN-juh-ghitt) has the same numbers but the opposite sign in the middle. So not only is the conjugate of , but is the conjugate of .
Also, conjugates don't have to be two-term expressions with radicals in each of the terms. In fact, any two-term expression can have a conjugate:
is the conjugate of
is the conjugate of
is the conjugate of
To create the conjugate, all you do is flip the sign in the middle. Everything else remains the same.
-
What is the conjugate of ?
In this case, I'm finding the conjugate for an expression in which only one of the terms has a radical. That's fine. The process is the same, regardless; namely, I flip the sign in the middle. Since they gave me an expression with a "plus" in the middle, the conjugate is the same two terms, but with a "minus" in the middle:
-
Find the conjugate of
This time, the radical is in the first of the two terms, and there's a "minus" in front of the first term. That's fine. I'll leave the first "minus" alone, because I don't change any but the middle sign; I'll flip the second "minus" in the middle to a "plus":
When we multiply conjugates, we are doing something similar to what happens when we multiply to a difference of squares; namely:
a2 − b2 = (a + b)(a − b)
When we multiply the factors a + b and a − b, the middle "ab" terms cancel out:
The same thing happens when we multiply conjugates:
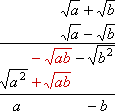
We will see shortly why this matters. To get to that point, let's first take a look at fractions containing radicals in their denominators.
Dividing by Square Roots
Just as we can swap between the multiplication of radicals and a radical containing a multiplication, so also we can swap between the division of roots and one root containing a division.
-
Simplify:
![sqrt[ 8 / 2 ]](radicals/rad064.gif)
I can simplify this by working inside, and then taking the square root:
![sqrt[ 8 / 2 ] = sqrt[4] = 2](radicals/rad065.gif){kind=small}
...or else by splitting the division into two radicals, simplifying, and cancelling:
![sqrt[8/2] = sqrt[8] / sqrt[2] = (2 sqrt[2]) / sqrt[2] = 2](radicals/rad066.gif)
Either way, my final answer is the same.
-
Simplify:
![sqrt[ 3 / 25 ]](radicals/rad068.gif)
I can see that the denominator contains a perfect square, but the numerator contains a prime number. So simplification will be easier if I split the radical containing a fraction into a fraction containing radicals:
![sqrt[ 3 / 25 ] = sqrt[3] / sqrt[25] = sqrt[3] / 5](radicals/rad069.gif){kind=small}
© 2024 Purplemath, Inc. All right reserved. Web Design by 