Reference Angles
Purplemath
In the previous section, we found the first-circle angle equivalents for given angle measures. Another thing we can do with angle measures, even those whose measures are in the first go-around, is to find what is called the "reference" angle.
The reference angle is the angle that the given angle makes with the x-axis. Regardless of where the angle ends (that is, regardless of the location of the terminal side of the angle), the reference angle measures the closest distance of that terminal side to the x-axis.
Content Continues Below
When finding reference angles, it can be helpful to keep in mind that the positive x-axis is 0° (and 360° or 0 radians (and 2π radians); the positive y-axis is 90° or radians; the negative x-axis is 180° or π radians; and the negative y-axis is 270° or radians.
Let's get started with an easy example. The angle with measure 30° would graph like this:

For graphing, the angle's initial side is the positive x-axis; its terminal side is the green line, because angles are drawn going anti-clockwise. The curved green line shows the given angle.
Even before having drawing the angle, I'd have known that the angle is in the first quadrant because 30° is between 0° and 90°. The reference angle, shown by the curved purple line, is the same as the given angle.
The angle 150°, obviously, is not the same as the angle 30°; it's bigger, and its terminal side is in the second quadrant (because 150° is between 90° and 180°). However, that terminal side is only 30° from the negative x-axis, as you can see by the purple line in the drawing:
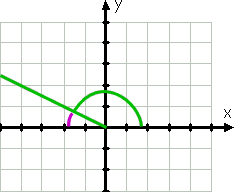
Since the terminal side of the 150° is only thirty degrees from the (negative) x-axis (being thirty degrees less than 180°, which is the negative x-axis), then the reference angle (again shown by the curved purple line) is 30°.
Continuing around counter-clockwise, we can graph 210°. This angle's terminal side, because 210° is between 180° and 270°, is in the third quadrant, and this side is closest to the negative x-axis. Because 210 is thirty more than 180, then this angle's terminal side is 30° past (that is, below) the negative x-axis.
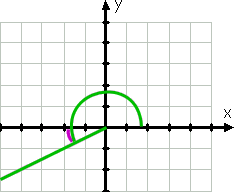
Therefore, the reference angle is, again, 30°.
I'll bet you can guess what would be the reference angle for 330°. Since 330 is thirty less than 360, and since 360° = 0°, then the angle 330° is thirty degrees below (that is, short of) the positive x-axis, in the fourth quadrant. So its reference angle is 30°.
Affiliate
Notice how this last calculation was done. I didn't have a graph. I just did the arithmetic in my head. You should draw graphs for as long as you need the help, but don't be afraid to start relying on the arithmetic. Once you get the hang of this, it's really pretty straightforward.
Note: Because the reference angle always measures the (positive) distance from the x-axis, it can also be viewed as being the first-quadrant equivalent angle. In other words, for each of the examples above, if my textbook defined "reference angle" as "the first-quadrant angle with the same distance from the x-axis", then the purple "reference angle" line (the curved purple line, plus a terminal side) would have been drawn in the first quadrant.
Either way, the value for the reference angle will always be the same. But if you are required to draw a picture showing the reference angle, make sure you draw it in the location that's regarded as "correct" for your class.
-
Find the reference angle for 1500°.
I'll grab my calculator and do the division by 360° for "once around":
1500 ÷ 360 = 4.16666...
So there are four cycles, plus a little. How much is just the four cycles?
4 × 360 = 1440
That means that the left-over portion (the 0.16666... above) represents another sixty degrees. This is smaller than ninety degrees, so the terminal side of the angle is to the right of the positive y-axis. Then the reference angle is in the first quadrant and is equal to:
60°
Content Continues Below
-
Find the first-quadrant reference angle for 954°, and draw both angles on the same axis system.
I'll start by reducing this angle. How many cycles fit within this angle?
954 ÷ 360 = 2.65
Two cycles fit within the angle. How much of the angle's measure do those two cycles take up?
360 × 2 = 720
Then how much is left over?
954 − 720 = 234
The negative x-axis is 180°, and the negative y-axis is 270°. This angle is between those values, so it's in the third quadrant, and will be closest to the negative x-axis.
How close? It'll be the distance between the terminal side of the reduced angle and the negative x-axis:
234 − 180 = 54
Then the reference angle is:
54°
...and the drawing is:
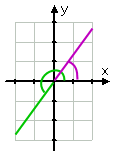
Affiliate
Notice how I drew the reduced angle (being the original angle, less two cycles) in green, and then I drew the first-quadrant reference angle in purple. When you're doing drawings that contain two (or more) distinct pieces of information, it can be helpful to have colored pencils on hand. Yes, I used colored pencils in college.
-
Find the reference angle for radians.
Advertisement
Okay, this is in radians. So I'll need to think in terms of 0 radians and 2π radians for the positive x-axis, and π radians for the negative x-axis.
The angle they've given me is radians. Doing the division to convert the fractional form to decimal form (and ignoring the π for the moment), I get:
16 ÷ 5 = 3.2
In other words, radians is equal to 3.2π radians. One cycle is 2π radians, so this is a bit more than half-again as much as one cycle. In other words, this angle goes a little past the negative x-axis:
3.2π − 2π = 1.2π
But how far is the terminal side from the negative x-axis?
I can figure this out by subtracting the angle measure of the negative x-axis from my reduced angle:
1.2π − 1π
= 0.2π
= (1/5)π
This gives me the distance between the terminal side of the (reduced) angle and the (negative) x-axis in radians. The reference angle is:
radians
-
Find the reference angle for radians.
Since is less than 2 but more than , then this angle is in the fourth quadrant, between radians and 2π radians. Then it's closest to the positive x-axis. But how close? I'll subtract to find out:
Then the reference angle is:
radians
Whether you're working in degrees or in radians, as long as you know the angle measures for the positive and negative portions of the x-axis, you can reduce the angle (if needed) and then do subtractions to get the reference angle. If you're not sure of your work, you can draw the picture to be sure. But if you're still needing to draw pictures when the test is coming up, try doing some extra practice, because the test is going to assume that you don't need the time to draw the pictures.
You can use the Mathway widget below to practice finding the median. Try the entered exercise, or type in your own exercise. Then click the button and select "Find the Reference Angle" to compare your answer to Mathway's.
Please accept "preferences" cookies in order to enable this widget.
(Click "Tap to view steps" to be taken directly to the Mathway site for a paid upgrade.)
URL: https://www.purplemath.com/modules/radians3.htm
Select a Course Below
Standardized Test Prep
Homeschool Math
© 2024 Purplemath, Inc. All right reserved. Web Design by ![]()




