Long Polynomial Division
Purplemath
Unlike the examples on the previous page, nearly all polynomial divisions do not "come out even"; usually, you'll end up with a remainder.
-
Divide 3x3 − 5x2 + 10x − 3 by 3x + 1
I start with the long-division set-up:
Looking only at the leading terms, I divide 3x3 by 3x to get x2. This is what I put on top:
I multiply this x2 by the 3x + 1 to get 3x3 + 1x2, which I put underneath:
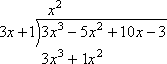
Then I change the signs, add down, and remember to carry down the "+10x − 3" from the original dividend, giving me a new bottom line of −6x2 + 10x − 3:
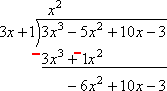
Dividing the new leading term, −6x2, by the divisor's leading term, 3x, I get −2x, so I put this on top:
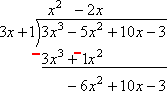
Then I multiply −2x by 3x + 1 to get −6x2 − 2x, which I put underneath. I change signs, add down, and remember to carry down the "−3 from the dividend:
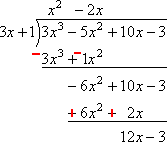
My new last line is "12x − 3. Dividing the new leading term of 12x by the divisor's leading term of 3x, I get +4, which I put on top. I multiply 4 by 3x + 1 to get 12x + 4. I switch signs and add down. I end up with a remainder of −7:
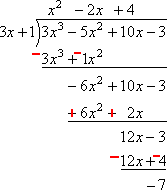
This division did not come out even. What am I supposed to do with the remainder?
Think back to when you did long division with plain numbers. Sometimes there would be a remainder; for instance, if you divide 132 by 5:
...there is a remainder of 2. Remember how you handled that? You made a fraction, putting the remainder on top of the divisor, and wrote the answer as "twenty-six and two-fifths":
The first form, without the "plus" in the middle, is how "mixed numbers" are written, but the meaning of the mixed number is actually the form with the addition.
We do the same thing with polynomial division. Since the remainder in this case is −7 and since the divisor is 3x + 1, then I'll turn the remainder into a fraction (the remainder divided by the original divisor), and add this fraction to the polynomial across the top of the division symbol. Then my answer is this:
Warning: Do not write the polynomial "mixed number" in the same format as numerical mixed numbers! If you just append the fractional part to the polynomial part, this will be interpreted as polynomial multiplication, which is not what you mean!
Note: Different books format the long division differently. When writing the expressions across the top of the division, some books will put the terms above the same-degree term, rather than above the term being worked on. In such a text, the long division above would be presented as shown here:
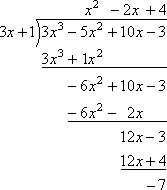
The only difference is that the terms across the top are shifted to the right. Otherwise, everything is exactly the same; in particular, all the computations are exactly the same. If in doubt, use the formatting that your instructor uses.
You may be wondering how I knew to stop when I got to the −7 remainder. It's much like how you knew when to stop when doing the long division (before you learned about decimal places). Once you got to something that the divisor was too big to divide into, you'd gone as far as you could, so you stopped; whatever else was left, if anything, was your remainder. The same goes for polynomial long division. The −7 is just a constant term; the 3x is "too big" to go into it, just like the 5 was "too big" to go into the 2 in the numerical long division example above. Once you get to a remainder that's "smaller" (in polynomial degree) than the divisor, you're done.
-
Divide 2x3 − 9x2 + 15 by 2x − 5
First off, I note that there is a gap in the degrees of the terms of the dividend: the polynomial 2x3 − 9x2 + 15 has no x term. My work might get complicated inside the division symbol, so it is important that I make sure to leave space for a x-term column, just in case. (This is like a zero in, say, the hundreds place of the dividend holding that column open for subtractions under the long-division symbol.) I can create this space by turning the dividend into 2x3 − 9x2 + 0x + 15.
(This is a legitimate mathematical step. I've only added zero, so I haven't actually changed the value of anything.)
Now that I have all the "room" I might need for my work, I'll do the division. I start, as usual, with the long-division set-up:
Dividing 2x3 by 2x, I get x2, so I put that on top. Then I multiply the x2 by the 2x − 5 to get 2x3 − 5x2, which I put underneath. Then I change the signs, add down, and carry down the 0x + 15 from the original dividend. This gives me −4x2 + 0x + 15 as my new bottom line:
Dividing −4x2 by 2x, I get −2x, which I put on top. Multiplying this −2x by 2x − 5, I get −4x2 + 10x, which I put underneath. Then I change the signs, add down, and carry down the +15 from the previous dividend. This gives me −10x + 15 as my new bottom line:
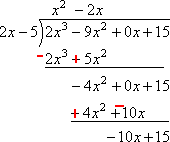
Dividing −10x by 2x, I get −5, which I put on top. Multiplying −5 by 2x − 5, I get 10x + 25, which I put underneath. Then I change the signs and add down, which leaves me with a remainder of −10:
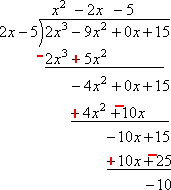
I need to remember to add the remainder to the polynomial part of the answer:
-
Divide 4x4 + 1 + 3x3 + 2x by x2 + x + 2
First, I'll rearrange the dividend, so the terms are written in the usual order:
4x4 + 3x3 + 2x + 1
I notice that there's no x2 term in the dividend, so I'll create one by adding a 0x2 term to the dividend (inside the division symbol) to make space for my work.
Then I'll do the division in the usual manner. Dividing the 4x4 by x2, I get 4x2, which I put on top. Then I multiply through, and so forth, leading to a new bottom line:
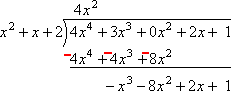
Dividing −x3 by x2, I get −x, which I put on top. Then I multiply through, etc, etc:
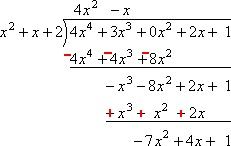
Dividing −7x2 by x2, I get −7, which I put on top. Then I multiply through, etc, etc:
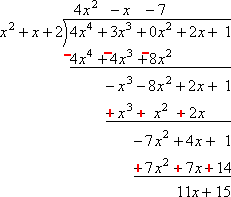
And then I'm done dividing, because the remainder is linear (11x + 15) while the divisor is quadratic. The quadratic can't divide into the linear polynomial, so I've gone as far as I can.
Then my answer is:
To succeed with polyomial long division, you need to write neatly, remember to change your signs when you're subtracting, and work carefully, keeping your columns lined up properly. If you do this, then these exercises should not be very hard; annoying, maybe, but not hard.
© 2024 Purplemath, Inc. All right reserved. Web Design by 