Place Value: Decimal Numbers

Think back to our fingers-and-marbles model. At each step going to the left, our units grew larger by a factor of 10. We started with 1s (being our fingers), and went to 10s (being the marbles in the first pile, standing for one instance of "all-my-fingers") and then 100s (being the marbles in the second pile, standing for ten times of "all-my-fingers").

So, at each stage leftward, we multiplied by 10. On the other hand, when we go off to the right (that is, when we go into smaller-than-one units), we divide by 10.

To picture this process with the smaller numbers, let's imagine that we're counting packs of candy bars. Each pack of candy bars contains ten individual bars, and each individual bar is formed so as to break neatly into ten pieces. How would we count single bars, or parts of bars, in our fingers-and-marbles model?

Let's say we counted up thirteen packs and two single bars. Remember that "one pack" is our unit; each finger counts one pack of bars.

Each pack is ten bars, so two single bars would be two parts of our "unit"; that is, they're two parts out of the ten parts of what we're calling "1". Using marbles, this time to the right of our hands, to indicate "parts of a pack", we'd count like this:

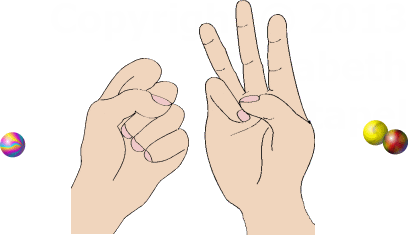
The picture above shows "ten packs (being the marble at the left), plus another three packs (being the three fingers), plus two parts of a pack (being the two marbles to the right)", or 10 + 3 + ²/₁₀.

When writing this out in numbers, we could use a line to divide between the whole numbers (in this case, the one 10 and the three 1s) and the parts (in this case, the two 1/10s):

...but the vertical dividing line looks too much like the digit "1", so instead, let's use a dot:

This dot is called the "decimal point". The number above can be read in either of two ways: "thirteen point two" or "thirteen and two tenths". In this case, since we're counting packs of candy bars, the number stands for "thirteen and two tenths packs of candy bars".

Note: The use of "and" in this context is important. It indicates that you have a whole-number part, "and" also a smaller-than-one part. The "and" goes between the two parts.

---

Now let's say we got hungry and ate three pieces of one of the loose bars. One bar is one-tenth of a pack, and the seven remaining pieces are seven-tenths of a bar, so those pieces are seven-tenths of something that is one-tenth of a unit, or (7/10) × (1/10) = 7/100 of a pack. So, after snacking, we have:

That is, we have thirteen point one seven (or thirteen and seventeen hundredths) packs of candy bars left.

---

Just as we can count bigger and bigger numbers by using digits further and further to the left, so also we can count smaller and smaller numbers by using digits further and further to the right. Each place further to the right counts pieces that are 1/10 as big as the piece at the previous step, and the names are similar to the whole-number names, as you can see in the rows of the table below:

When the numbers get really small (that is, really far to the right of the dot), the names for those numbers get really big, so people usually switch to the "point" way of reading the numbers; it's easier.

---

When we're working with numbers smaller than the whole numbers (that is, when we're working with numbers with the "dot" or "decimal point" in them), we have to use zeroes as placeholders after the decimal point. For instance, the fraction "7/100" is written in decimal form as "0.07". If we didn't include that zero between the dot and the 7, we'd be saying "7/10", which wouldn't be what we'd meant. Also, for however many digits we have to the right of the decimal point, that's how many "decimal places" we have.

How are big- and small-number place names related?

Take a close look at the correspondence between the numbers of zeroes in the "big units" expressions in the table above and the numbers of decimal places in the "small units" expressions; if you count them up, you'll see that they match. For instance, one thousand has three zeroes, and one thousandth has three decimal places.

---

To figure out what "place" we're at when looking at a decimal-places number, we count up the digits after the decimal point. Whatever is the number of decimal places that we have, that's the number of zeroes for the number matching the decimal "place" that we're at. For instance, the number 83.295 has three digits after the decimal point (those digits begin the 2, 9, and 5), so the number has three decimal places. The number with 1 followed by three zeroes is 1,000: one thousand. That means that the 0.295 portion of the number stands for "two hundred ninety-five thousandths", and the entire number 83.295 stands for "eighty-three and two hundred ninety-five thousandths".

How is "and" used in decimal numbers in "real life"?

If you've ever written a check, or seen one filled out, you will have seen that the amount of the check is written in two places: in numerical characters inside a box to the right, and as words on the second line of the check. On the line where the amount is written out in words, there is an "and" between the whole-dollar (or the whole-rand, or the whole-naira, or the whole pretty much any other modern currency) portion and the cents portion. This is the only place where "and" is appropriate when saying or spelling out numbers!

(Yes, pretty much every currency has by now been "decimalized". The main currency unit will go by different names in different countries — guilders, pesos, rials, euros, kwacha, whatever — but they all are subdivided into one hundred of a smaller unit — fils, kopeks, naya paisas, metonnyas, satangs, whatever.)

---

• Express "47.3692" (a) in expanded notation and (b) in words.

Again, note the "and" that went between the whole-number part (being the 47) and the decimal-places portion (being the 3692). This is the only proper place for an "and" within a number name.

---

• Given the number 16.875, state (a) the value of the 5 and (b) the digit in the tenths place.