Multiplying and Dividing Negative Numbers
Purplemath
Turning from addition and subtraction with negative numbers, we now turn to multiplying and dividing negative numbers.
So how do we do multiplication and division with negatives? Actually, we've already covered the hard part!
Content Continues Below
When we were subtracting a negative number, we saw that the minus (the subtraction) of a minus (a negative number) was a plus (that is, it was the same as adding a positive number). In other words, you already know the rules for multiplying with negative numbers. To refresh:
Let's use, as our model, a hot-air balloon; turning on the burner is "positive" and causes the balloon to rise; adding more sand bags (we're pretending that the bags are hovering in the air nearby for us to grab) is "negative" and causes the balloon to sink. Then the rules for multiplying with negatives are:
plus times plus is a plus
(firing the burner causes the balloon to rise)
minus times plus is a minus
(turning the burner off causes the balloon to sink)
plus times minus is a minus
(adding sand bags causes the balloon to sink)
minus times minus is a plus
(dropping sand bags causes the balloon to rise)
The sign rules work the same way for division; just replace "times" with "divided by". Here is an example of the rules in division:
Or, remembering that fractions are division:
Some people like to think of negative numbers in terms of debts. So, for instance, if you owe $10 to each of six people, then your total debt would be 6 × $10 = $60. In this context, getting a negative answer makes sense. But in what context could dividing a negative by a negative (and getting a positive) make any sense?
What is a real-world example of "minus divided by minus is plus"?
Think about having a snack at a café. When you go to pay, the kid has trouble running your debit card. He swipes it six times before finally returning the card to you. When you get home, you check your bank account online. You can tell from the amount that, yes, he actually charged you way more than once. Some portion of that total debit (being a negative on your account) is wrong.
You want to confirm the number of over-charges before you call your bank to correct the situation. How can you figure this out? You can divide the entire amount (let's say, $76.02) by the amount shown on your receipt (say, $12.67), which is the amount of one charge. Each charge is a minus on your account, so the math is:
(− $76.02) ÷ (− $12.67) = 6
So there were indeed six charges in total. The number of charges, 6, being the counting-up of the number of events, should be positive. In this real-world context, dividing a minus by a minus and getting a plus makes perfect sense. (And now you know to direct customer service to cancel exactly five of the charges.)
(If you're interested, TheMathDoctors have articles with real-world examples and proper mathematical proofs of "minus of a minus is a plus",.)
Affiliate
Advertisement
You may notice that many people, when working with the minus of a minus, will "cancel off" pairs of minus signs. They are taking advantage of the fact that "minus times minus is plus".
For instance, suppose you have (−2)(−3)(−4). Any two negatives, when multiplied together, become one positive. So pick any two of the multiplied (or divided) negatives, and "cancel" their signs by drawing vertical lines through the "minus" signs, turning them into "plus" signs.
- Simplify (−2)(−3)(−4)
I'll start by cancelling off one pair of "minus" signs. Then I'll multiply as usual.
(−2)(−3)(−4)
= (−2)(−3)(−4)
= (+6)(−4)
= −24
If you're given a long multiplication with negatives, just cancel off "minus" signs in pairs:
- Simplify (−1)(−2)(−1)(−3)(−4)(−2)(−1)
The first thing I'll do is count up the "minus" signs. One, two, three, four, five, six, seven. So there are three pairs that I can cancel off, with one left over. As a result, my final answer should be negative. If I come up with a positive result, I'll know I've done something wrong.
(−1)(−2)(−1)(−3)(−4)(−2)(−1)
= (−1)(−2)(−1)(−3)(−4)(−2)(−1)
= (+1)(+2)(−1)(−3)(−4)(−2)(−1)
= (1)(2)(−1)(−3)(−4)(−2)(−1)
= (1)(2)(+1)(+3)(−4)(−2)(−1)
= (1)(2)(1)(3)(−4)(−2)(−1)
= (1)(2)(1)(3)(+4)(+2)(−1)
= (1)(2)(1)(3)(4)(2)(−1)
= (2)(3)(4)(2)(−1)
= 48(−1)
= −48
I got a negative answer, so I know my sign is correct.
Content Continues Below
Here's another example, showing the same cancellation process in a division context:
How do you take a "minus" through parentheses?
To take a "minus" through a set of parentheses, you distribute the "minus" onto every term inside the parentheses, just as you would take a number through onto every term inside parentheses.
The major difficulty that people have with negatives is in dealing with parentheses; particularly, in taking a negative through parentheses. The usual situation is something like this:
−3(x + 4)
If you had the expression 3(x + 4), you would know to distribute the 3 over the parentheses:
3(x + 4) = 3(x) + 3(4) = 3x + 12
The same rules apply when you're dealing with negatives. If you have trouble keeping track, use little arrows:
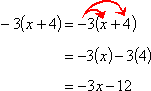
- Simplify 3(x − 5)
I need to take the 3 through the parentheses:
3(x − 5)
3(x) + 3(−5)
3x − 15
- Simplify −2(x − 3)
Here, I'll be taking a "minus" through the parentheses; in particular, I'll be distributing the − sign, along with its 2, onto the x and the minus of the 3.
−2(x − 3)
−2(x) − 2(−3)
−2x + 2(+3)
−2x + 6
Affiliate
Notice how I was careful to keep track of the signs inside the parentheses. The "minus" sign was kept with the 2 by use of another set of parentheses. Do not be shy about using grouping symbols to keep your intended meaning clear, both to the grader and to yourself.
Another thing that can cause difficulty, related to the previous one, is with subtracting a parentheses. You can keep track of the subtraction sign by converting the subtraction of the parenthetical to a multiplication by −1:
- Simplify 4 − (2 + x)
I'll start by writing a little 1 in front of the parentheses. Then I'll draw arrows from this 1 to the terms inside the parentheses, to remind myself of what I'm needing to do.
4 − (2 + x)
= 4 − 1(2 + x)
= 4 −1(2) −1(x)
= 4 − 2 − 1x
= 2 − 1x
= 2 − x
Don't be afraid to write in that little 1 and draw in those little arrows. You should do whatever you need to do to keep your work straight and to consistently get the right answers.
- Simplify 6 − (3x − 4[1 − x])
I'll work from the inside out, simplifying first inside the inner grouping symbols, according to the Order of Operations. So the first thing I'll do is take the −4 through the brackets. Then I'll simplify; I'll continue by putting a 1 in front of the parentheses and, to help me keep track of that −1 that I'll be distributing, I'll draw my little arrows.
6 − (3x − 4[1 − x])
= 6 − (3x − 4[1] − 4[x])
= 6 − (3x − 4 + 4x)
= 6 − (7x − 4)
= 6 − 1(7x − 4)
= 6 − 1(7x) − 1(−4)
= 6 − 7x + 4
= −7x + 10
- Simplify
This one is tricky. They're having me subtract a fraction. I need to combine the fractions, which means combining the numerators. To make sure that I don't lose track of exactly what that "minus" means (namely, that I'm minus-ing the whole numerator of the second fraction, not just the x at the beginning of that numerator), I'll convert the minus to a plus of a −1:
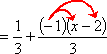
Note that I converted from subtracting a fraction to adding a negative one times a fraction. It is very easy to lose the minus when you're adding messy polynomial fractions like this. The most common mistake is to put the minus on the x and forget to take it through to the −2. Take particular care with fractions.
Also, the answer above could have been stated as . Either form should be acceptable.
(For extra practice simplifying with parentheses, try here.)
URL: https://www.purplemath.com/modules/negative3.htm
Select a Course Below
Standardized Test Prep
Homeschool Math
© 2024 Purplemath, Inc. All right reserved. Web Design by ![]()