Extracting Information from the Hyperbola's Equation

Since the y part of the equation is added, then the center, foci, and vertices will be above and below the center, on a line paralleling the y-axis, rather than side by side.

Looking at the denominators, I see that a² = 25 and b² = 144, so a = 5 and b = 12.

The equation c² = a² + b² tells me that

(Recall that c is a length, so its value must be positive.)

Then the eccentricity is given by:

Since x² = (x − 0)² and y² = (y − 0)², then the center is at (h, k) = (0, 0).

The vertices and foci are above and below the center, so the foci are at (0, −13) and (0, 13), and the vertices are at (0, 5) and (0, −5).

Because the y part of the equation is dominant (being added, not subtracted), then the slope of the asymptotes has the a on top, so the slopes will be .

For my sketch, I start with the center, and draw the asymptotes through it, using dashed lines:

Then I draw in the vertices, and rough in the graph, rotating the paper as necessary and "eye-balling" for smoothness:

Then I draw in the final graph as a neat, smooth, heavier line:

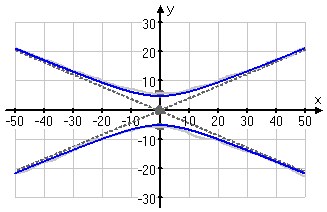
And the rest of my answer is:

Since the x part is added, then a² = 16 and b² = 9, so a = 4 and b = 3.

Also, this hyperbola's foci and vertices are to the left and right of the center, on a horizontal line paralleling the x-axis.

From the equation, clearly the center is at (h, k) = (−3, 2).

Since the vertices are a = 4 units to either side, then they are at the points (−7, 2) and at (1, 2).

The equation a² + b² = c² gives me:

Then the foci, being 5 units to either side of the center, must be at (−8, 2) and (2, 2).

Since the a² went with the x part of the equation, then a is in the denominator of the slopes of the asymptotes, giving me:

Keeping in mind that the asymptotes go through the center of the hyperbola, the asymptes are then given by the following straight-line equations:

Putting it all together, my answer is:

To find the information I need, I'll first have to convert this equation to vertex form by completing the square. The following are my steps in that process, starting with grouping the x- and y-containing terms together, and moving the loose number to the other side of the "equals" sign:

Now that I've converted the equation from multiplied-out form to center-vertex form, I can start extracting information.

The center is at (h, k) = (−5, −3).

Since the x-part of the equation is added, then the center, foci, and vertices lie on a horizontal line paralleling the x-axis. From the denominators, I get a² = 25 and b² = 20, so a = 5 and . The equation a² + b² = c² gives me:

The slopes of the two asymptotes will be , so the asymptotes' equations are given by:

Then my complete answer is:

First, I'll need to put this equation into conics form, by completing the square. (In this case, all I'll need to do is divide through by 1872.)

So clearly the center of this hyperbola is at the origin; also, a² = 144 and b² = 52. Since the y-part is added in the equation, then the branches are indeed upper and lower; I'll be working with the lower branch.

The tip of the gnomon (NOH-munn, being the stick or pointer that casts the shadow) will be at (well, floating the air above) the focus of the lower branch; the tip of its shadow *at noon* will be at the vertex of the lower branch. So I need to find the lower vertex and the lower focus. For this, I'll need the value of c.

The vertex will be a units below the center; the focus will be c units below the center. So the tip of the shadow will be at (0, −a) = (0, −12), and the gnomon will be at (0, −c) = (0, −14).

But they didn't ask me for the algebraic locations; they asked me for the location of the shadow's tip relative to the location of the gnomon. And, looking at the points, I can see that my answer should be: